INCA

Effectivity and efficiency of selective frequency damping for the computation of unstable steady-state solutions

J. Casacuberta, K.J. Groot, H.J. Tol, S. Hickel (2018)
Journal of Computational Physics 375: 481-497. doi: 10.1016/j.jcp.2018.08.056

Selective Frequency Damping (SFD) is a popular method for the computation of globally unstable steady-state solutions in fluid dynamics. The approach has two model parameters whose selection is generally unclear. In this article, a detailed analysis of the influence of these parameters is presented, answering several open questions with regard to the effectiveness, optimum efficiency and limitations of the method.

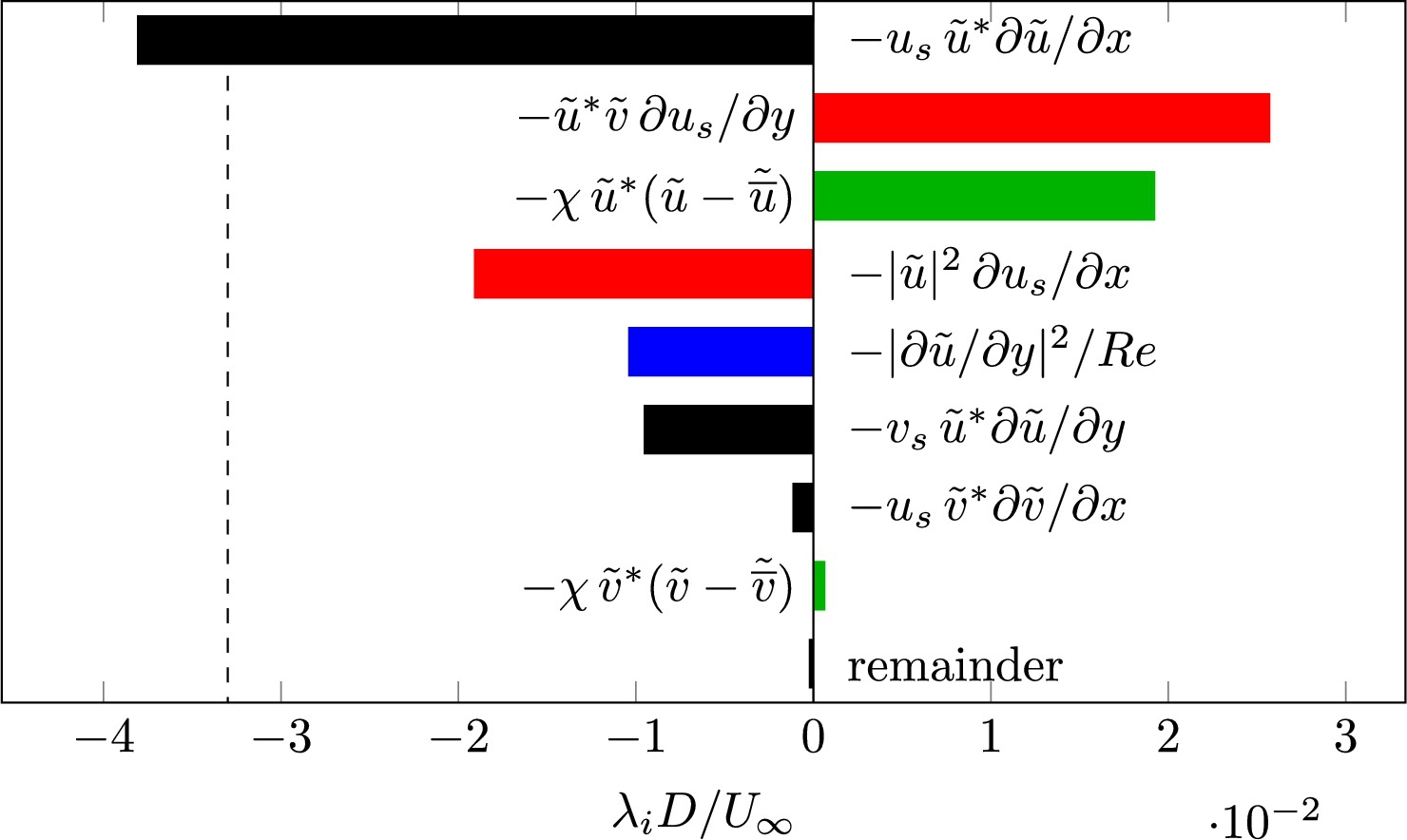
In particular, we show that SFD is always capable of stabilising a globally unstable systems ruled by one unsteady unstable eigenmode and derive analytical formulas for optimum parameter values. We show that the numerical feasibility of the approach depends on the complex phase angle of the most unstable eigenvalue. A numerical technique for characterising the pertinent eigenmodes is presented. In combination with analytical expressions, this technique allows finding optimal parameters that minimise the spectral radius of a simulation, without having to perform an independent stability analysis. An extension to multiple unstable eigenmodes is derived. As computational example, a two-dimensional cylinder flow case is optimally stabilised using this method. We provide a physical interpretation of the stabilisation mechanism based on, but not limited to, this Navier–Stokes example.

 

Controlled and uncontrolled evolution of residual and controlled and uncontrolled eigenspectrum (a) SFD residual  (solid lines) using χ = 0.5 and Δ = 3. Fits to exponential parts (dashed lines). Unleash times (yellow squares) at ϵR = 10−3 (black), 10−4 (blue), 10−5 (green), 10−6 (red). Location of null curvature in the linear range of the unleashed curves (red circles). (b) μ- (empty circles) and λ- (crosses) eigenspectrum from stability analysis computed with ϵR = 10−6 base flow.
λi-budget of the steady mode for the optimal control parameters (χopt,Δopt), λi eigenvalue (dashed line). Reynolds stress, viscous dissipation, advection and SFD model terms are coloured red, blue, black and green, respectively.

Publications