J. Matheis, H. Müller, C. Lenz, M. Pfitzner, S. Hickel (2016)
Journal of Supercritical Fluids 107: 422-432.
doi: 10.1016/j.supflu.2015.10.004
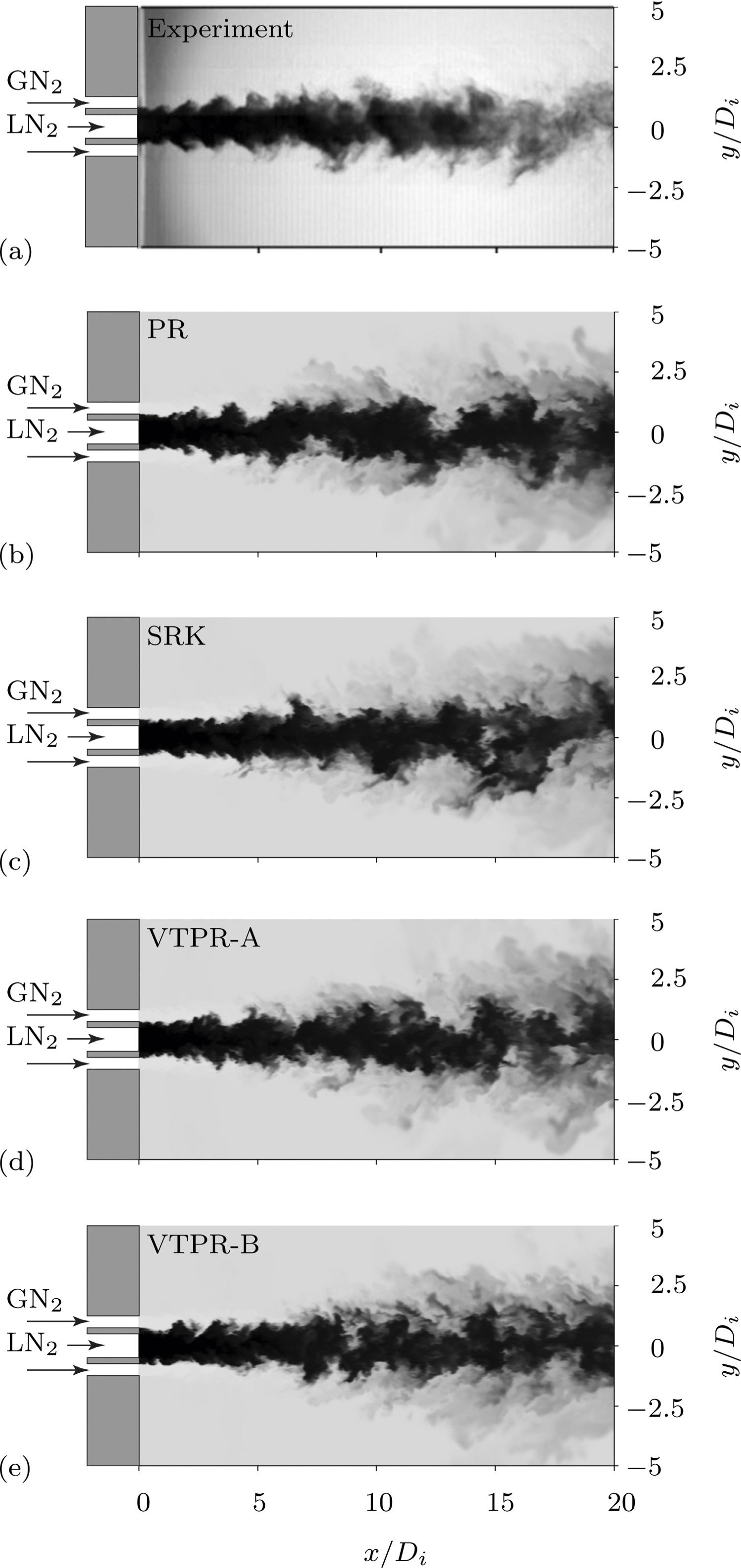
We report on recent developments within the field of real gas thermodynamics models with particular emphasis on volume translation methods for cubic equations of state. On the basis of the generalized form of a cubic equation of state, a mathematical framework for applying volume translations is provided, allowing for an unified and thermodynamically consistent formulation in the context of computational fluid dynamics simulations.
This generalized methodology is applied to two selected volume translation methods that were recently proposed: the method of Abudour et al. (Fluid Phase Equilibria, 2012) and the method of Baled et al. (Fluid Phase Equilibria, 2012). In particular, the consistent integration of both methods in the evaluation of the caloric properties is investigated, showing that for Abudour et al.'s method one has to apply numerical integration whereas an analytical solution exists for Baled et al.'s method. The results of both volume translations are compared with those of the untranslated equations of state as well as with reference data. The density predictions of Abudour et al.'s method proved to be superior to those of the other approaches, whereas we observe a decreased accuracy for caloric properties. To evaluate the applicability of both methods in the context of real-gas computational fluid dynamics simulations, we performed well-resolved large-eddy simulations for the coaxial injection of cryogenic and gaseous nitrogen into a supercritical nitrogen atmosphere. A qualitative comparison of the numerical results with the available experimental backlight image shows a good agreement and a comparison of different volume translation methods demonstrates the effect of the thermodynamic model on the flow field.